|Home|Atmospheric Lensing|Gravitational Lensing|GL Experiment|Examples|References|
During the elaboration of his theory of General Relativity, Einstein (1915)
predicted that a massive object curves the spacetime in its vicinity and
that any particle, massive or not (cf. the photons), will move along the
geodesics of this curved space. He showed that a light ray passing at a
distance ![]() from an object characterized by an axially symmetric mass
distribution M(
from an object characterized by an axially symmetric mass
distribution M(![]() ) (see Figure 1) will undergo a total deflection angle
) (see Figure 1) will undergo a total deflection angle
![]() , expressed in radian by means of the relation
, expressed in radian by means of the relation
![]()
where G stands for the gravitational constant and c for the velocity of light.
Adopting a given mass distribution (e.g. a constant mass ![]() in order
to characterize a point-like object, the disk of a spiral galaxy, etc.), it is
then trivial to construct an optical lens that deflects light rays
accordingly, thus enabling us to study very simply in the laboratory the
lensing properties of black holes, stars, quasars, galaxies, etc. as they
exist in the Universe. We discuss this in greater details in the next section.
in order
to characterize a point-like object, the disk of a spiral galaxy, etc.), it is
then trivial to construct an optical lens that deflects light rays
accordingly, thus enabling us to study very simply in the laboratory the
lensing properties of black holes, stars, quasars, galaxies, etc. as they
exist in the Universe. We discuss this in greater details in the next section.
Before discussing the optical gravitational lens experiment, we wish to state
that a sufficient condition for a gravitational lens to produce multiple
images of a background source is simply that its surface mass density
![]() exceeds the critical density
exceeds the critical density ![]() , which only depends
on the relative distances
, which only depends
on the relative distances ![]() ,
, ![]() and
and ![]() , between the observer (O),
the deflector (D) and the source (S) (cf. Equation (2.4)-(2.6)). It is also very
easy to show that in the case of a perfect alignment between a source, an
axially symmetric deflector and an observer, the latter will see the
background source as a ring of light (the so-called Einstein ring) which
angular radius
, between the observer (O),
the deflector (D) and the source (S) (cf. Equation (2.4)-(2.6)). It is also very
easy to show that in the case of a perfect alignment between a source, an
axially symmetric deflector and an observer, the latter will see the
background source as a ring of light (the so-called Einstein ring) which
angular radius ![]() is proportional to the square root of the mass of
the deflector (cf. Equation (2.7)). For those students who are very curious,
we propose hereafter the derivation of the quantities
is proportional to the square root of the mass of
the deflector (cf. Equation (2.7)). For those students who are very curious,
we propose hereafter the derivation of the quantities ![]() and
and
![]() (Exercise 2).
(Exercise 2).
2.1 Exercise 2: Condition for multiple imaging and angular radius of the Einstein ring
In the case of perfect alignment between a source (S), an axially symmetric deflector (D) and an observer (O) (see Fig. 1), we easily see that, with the exception of the direct ray propagating from the source to the observer, the condition for any other light ray to reach the observer is
![]()
as obtained from the direct application of the sine rule in the triangle SXO
and assuming that the angles ![]() and
and ![]() remain very
small. Of course, this will also be true if the real deflection angle
remain very
small. Of course, this will also be true if the real deflection angle ![]() since it will then be always possible to find
a light ray with a greater impact parameter
since it will then be always possible to find
a light ray with a greater impact parameter ![]() such that Eq. (2.2) is
fulfilled. Expressing the angle
such that Eq. (2.2) is
fulfilled. Expressing the angle ![]() between the direct ray and the
incoming deflected ray as
between the direct ray and the
incoming deflected ray as
![]()
and making use of Eqs. (2.1) and (2.2), we may thus rewrite the above condition as follows
![]()
i.e. the average surface mass density of the lens
![]()
evaluated within the impact parameter ![]() , must simply exceed the
critical surface mass density
, must simply exceed the
critical surface mass density ![]() , defined by
, defined by
![]()
Let us note that the latter quantity is essentially determined by the distances between the source, the deflector and the observer.
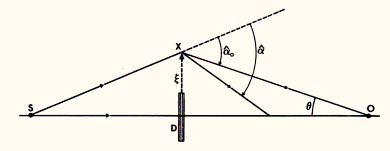
Figure 1: On the condition for an observer O to see a light ray from
a distant source S, deviated by a deflector D so that more than one image can
be seen. Note that O, D and S are perfectly co-aligned and that axial symmetry
is assumed. No scale is respected in this diagram
Although the above reasoning essentially applies to a static Euclidian space,
Refsdal (1966) has shown that it also remains valid for Friedmann,
Lemaître, Robertson, Walker (FLRW) expanding universe models, provided
that ![]() ,
, ![]() and
and ![]() represent angular size distances.
Adopting typical cosmological distances for the deflector
(redshift
represent angular size distances.
Adopting typical cosmological distances for the deflector
(redshift ![]() ) and the source (
) and the source ( ![]() ), we find that
), we find that
![]()
![]() .
.
Substituting M( ![]() ) and
) and ![]() in Eq. (2.5) with a typical mass M and a
radius R for the deflector, we have listed in Table 1 values for the ratio
in Eq. (2.5) with a typical mass M and a
radius R for the deflector, we have listed in Table 1 values for the ratio
![]() considering a star, a galaxy and a cluster of
galaxies located at various distances.
considering a star, a galaxy and a cluster of
galaxies located at various distances.

Table 1: Ratio of the average ![]() and critical
and critical ![]() surface mass densities, angular (
surface mass densities, angular ( ![]() ) and linear (
) and linear ( ![]() ) radii of the
Einstein ring for different values of the mass M, distance
) radii of the
Einstein ring for different values of the mass M, distance ![]() and
radius R of the deflector, assuming that
and
radius R of the deflector, assuming that ![]() (
( ![]()
We see that only stars and very compact, massive galaxies and galaxy clusters,
for which ![]() , constitute promising `multiple
imaging' deflectors.
, constitute promising `multiple
imaging' deflectors.
In the case of axial symmetry, it is clear that in the presence of an efficient deflector, an observer located on the symmetry axis will actually see a ring (the so-called 'Einstein ring', cf. Fig. 10) of light from a distant source. Combining Eqs. (2.1)-(2.3), the angular radius of this ring may be conveniently expressed as

We have also listed in Table 1 typical values of ![]() for different
types of deflectors located at various distances.
for different
types of deflectors located at various distances.
As we shall see in the optical gravitational lensing experiment, the value of
![]() derived above is very important because it can usually be
used to estimate the angular separation between multiple lensed images in
more general cases where the condition of a perfect alignment between the
source, deflector and observer is not fulfilled or even for lens mass
distributions which significantly depart from the axial symmetry. Knowing that
angular size distances are inversely proportional to the Hubble constant
derived above is very important because it can usually be
used to estimate the angular separation between multiple lensed images in
more general cases where the condition of a perfect alignment between the
source, deflector and observer is not fulfilled or even for lens mass
distributions which significantly depart from the axial symmetry. Knowing that
angular size distances are inversely proportional to the Hubble constant
![]() , observed image separations
, observed image separations ![]() can therefore
lead to the value of
can therefore
lead to the value of ![]() , or to the value of M times the Hubble
constant
, or to the value of M times the Hubble
constant ![]() , if the redshifts
, if the redshifts ![]() and
and ![]() are known. This is the simplest
and most direct astrophysical application of gravitational lensing. We see
from Table 1 that for a source and a lens located at cosmological
distances
are known. This is the simplest
and most direct astrophysical application of gravitational lensing. We see
from Table 1 that for a source and a lens located at cosmological
distances ![]() and
and ![]() ), the angle
), the angle
![]() can vary from micro-arcsec (stellar deflection) to arcsec
(galaxy lensing), and up to some tens of arcsec in the case of cluster lenses.
Let us also finally note that the condition (2.4) for a deflector to produce
multiple images of a lensed source turns out to be usually applicable, even
when there is no axial symmetry.
can vary from micro-arcsec (stellar deflection) to arcsec
(galaxy lensing), and up to some tens of arcsec in the case of cluster lenses.
Let us also finally note that the condition (2.4) for a deflector to produce
multiple images of a lensed source turns out to be usually applicable, even
when there is no axial symmetry.